(17.1-17.2)项目实战:多机lnmp部署
本文共 1826 字,大约阅读时间需要 6 分钟。
文章目录
安装tengine+php
main.sh将整个文件夹lnmp推到不同的服务器上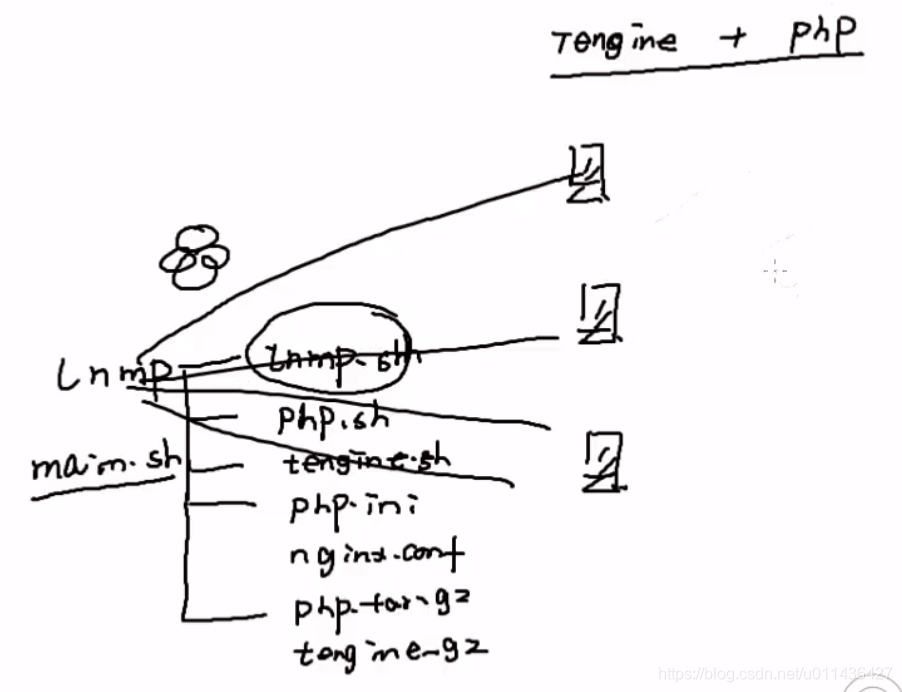
- 获取安装包
从172.16.8.100服务器获取php.tar.xz和tengine.tar.gz这两个包lftp 172.16.8.100cd source-softget php.tar.xz tengine.tar.gzby
-
yum install -y tree
tree可以看到目录结构,执行的是lnmp.sh脚本:它会调用一切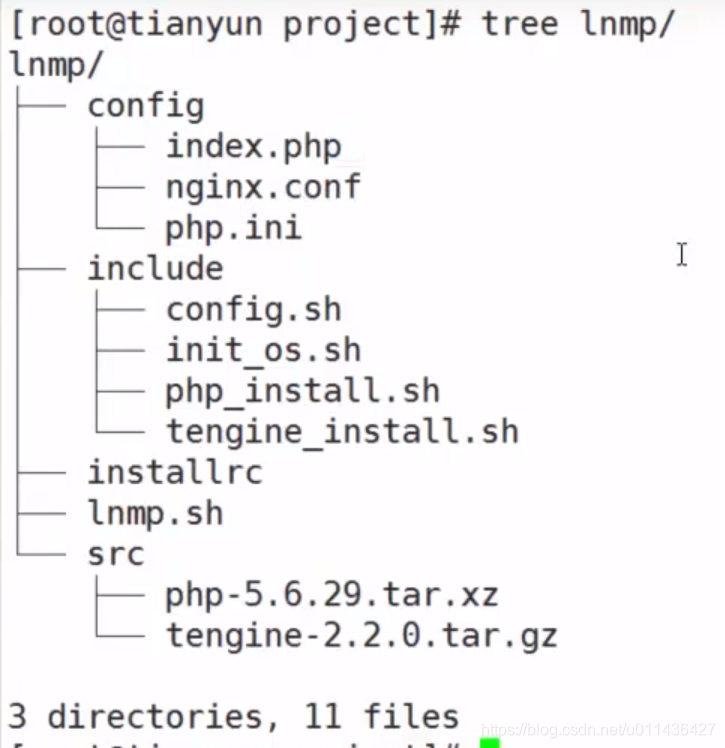
-
lnmp.sh脚本
#!/usr/bin/env bash#inmp installsoft_dir=`pwd`/srcconfig_dir=`pwd`/configcpus=`lscpu|awk '/^CPU\(s\)/{print $2}'`. installrc. include/init_os.sh. include/tengine_install.sh. include/php_install.sh. include/config.shinit_ostengine_installphp_installconfig - init.sh,该脚本放在include目录下面
#!/usr/bin/env bash#init os
- install_php.sh
#!/usr/bin/env bashphp_install(){ yum -y install libjpeg libjpeg-devel libpng libjpeg-devel freetype freetype-devel \ libxml2 libxml2-devel libcurl libcurl-devel libxslt-devel openssl-devel cd ${ soft_dir} tar xf ${ php_version} cd ${ php_version%.tar.xz} ./configure \ --prefix=${ php_prefix} \ --with-curl \ ... ... --enable-xml \ --enable-zip make -j ${ cpus} make install ##php-fpm配置文件:影响php处理php程序的性能,例如php进程数,最大连接数配置等 cp ${ php_prefix}/etc/php-fpm.conf.defaul ${ php_prefix}/etc/php-fpm.conf ##php配置文件:影响phph代码,eg:允许客户端最大上传文件的大小,php扩展功能是否可以连接MySQL,MEMcache等 cp php.ini-production /usr/local/php/lib/php.ini ##添加到init启动项 cp sapi/fpnfpm/init.d.php-fpm /etc/rc.d/init.d/php-fpm chmod a+x /etc/rc.d/init.d/php-fpm chkconfig --add php-fpm chkconfig php-fpm on service php-fpm start} - intstallrc
#!/usr/bin/env bash#installrctengine_version=tengine-2.2.0.tar.gzphp_version=php-5.6.29.tar.xztengine_prefix=/usr/local/tenginephp_prefix=/usr/local/phpnginx_prefix=/usr/local/nginx
- configure.sh
#!/usr/bin/env bashconfigure(){ ##放置替换文件时的提示,可以在cp前面加上转移\ \cp ${ config_dir}/nginx.conf ${ nginx_prefix}/conf/nginx.conf \cp ${ config_dir}/index.php ${ nginx_prefix}/html/index.php ${ nginx_prefix}/sbin/nginx /etc/init.d/php-fpm restart} 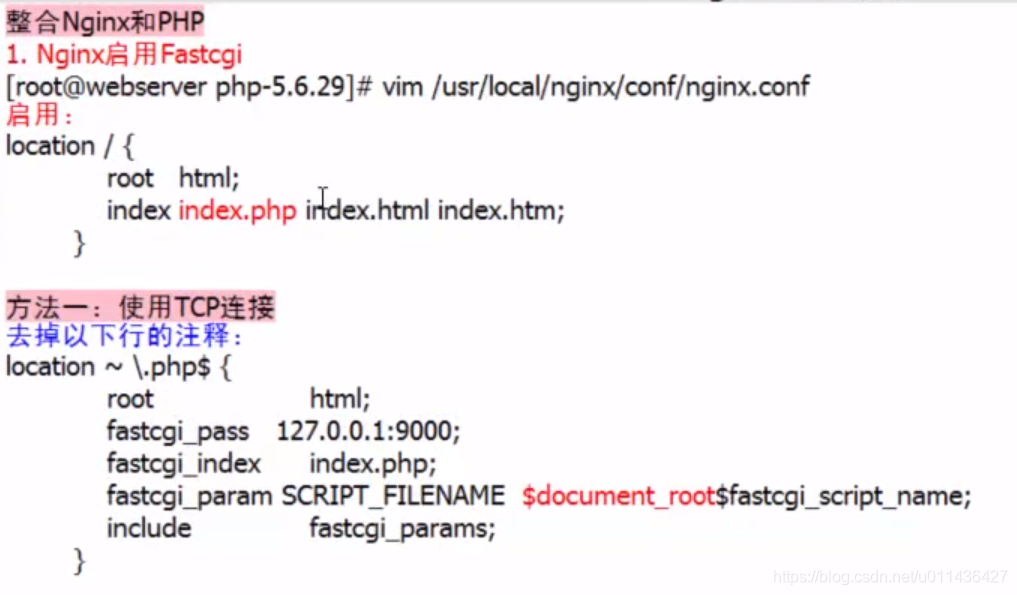

- include/index.php
转载地址:http://cjfzz.baihongyu.com/
你可能感兴趣的文章
mysql排序查询
查看>>
MySQL排序的艺术:你真的懂 Order By吗?
查看>>
MySQL排序的艺术:你真的懂 Order By吗?
查看>>
Mysql推荐书籍
查看>>
Mysql插入数据从指定选项中随机选择、插入时间从指定范围随机生成、Navicat使用存储过程模拟插入测试数据
查看>>
MYSQL搜索引擎
查看>>
mysql操作数据表的命令_MySQL数据表操作命令
查看>>
mysql操作日志记录查询_如何使用SpringBoot AOP 记录操作日志、异常日志?
查看>>
MySQL支持的事务隔离级别,以及悲观锁和乐观锁的原理和应用场景?
查看>>
mysql支持表情
查看>>
MySQL支撑百万级流量高并发的网站部署详解
查看>>
MySQL改动rootpassword的多种方法
查看>>
mysql数据分组索引_MYSQL之索引配置方法分类
查看>>
mysql数据取差,mysql屏蔽主外键关联关系
查看>>